What's the deal?

Over the past two years, #M323 teacher-leaders have designed several centers associated with Common Core State Standard 6.SPA.3. Below is one of my favorites, which I am attempting to revise for my #M221 pre-service teachers. Any feedback you are willing to provide would be appreciated.

=====

Data Set Deal

Rules

• Remove all the face cards and Jokers from a deck of cards;
• Deal out five cards, face down, to each player;

• Turn over exactly three cards;
• Determine the mode (color), median (number), and range (number) of the three cards;
• Other players check to see that your answers are correct [1 point per correct answer];
• Predict the mode (color), median (number), and range (number) of all five cards;

• Turn over another card;
• Determine the mode (color), median (number), and range (number) of the four cards;
• Other players check to see that your answers are correct [1 point per correct answer];
• Predict the mode (color), median (number), and range (number) of all five cards;

• Turn over the last card;
• Determine the mode (color), median (number), and range (number) of all five cards;
• Other players check to see that your answers are correct [1 point per correct answer];

• Check to see which of your predictions were correct [2 points per correct answer]; and

• The winner is the first one to 21 points.

Score Sheet

=====

Please leave any questions or suggestions in the comments. Thanks!

We're really going to get to do it, aren't we?

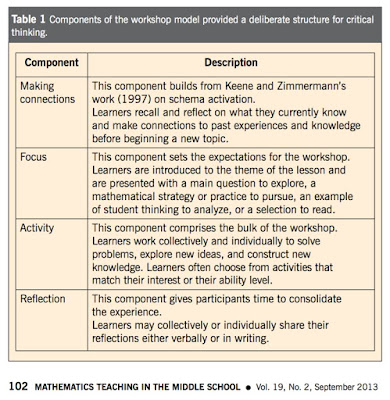
One of the projects the pre-service elementary teachers (math majors) that I teach worked on this semester was designing a 4th-grade statistics lesson to address 4.MD.B.4.

The teachers went through a design cycle to make the lesson. They ... 

• Built empathy by observing two fourth-grade classes;
• Defined the problem by developing a User/Needs/Insight statement;
• Brainstormed a variety of possible activities;
• Developed a prototype SAFARI Lesson;

• Tested the lesson by sharing it with the classroom teacher; and
• Revised it based on her feedback.

Three teachers co-taught the lesson in two different fourth-grade STEM classes. They made adjustment between the lessons based on what worked and what didn't. Afterwards, they reflected on the experience and shared the lesson with me. The lesson was so cool, I decided to make a few adjustments and use it in another class for pre-service elementary teachers (mostly non-math majors) that I teach. Here is the SAFARI Lesson that I taught.

Schema Activation - Prediction

Directions: "You have two sticky notes. On the green sticky, I want you to predict the number of seconds you think it would take you to write the alphabet from A to Z. On the purple sticky, I want you to predict how long it would take you to write the alphabet in reverse order from Z to A. You have a quarter of a minute. Go!"

Focus - 5.MD.B.2

Share lesson target: "We are going to make line plots to display data sets of measurements in fractions of a unit."

[Anticipated learner responses are in brackets.]

"Who thinks they can write the alphabet forward the fastest? [13 seconds or 2 letters per second] Who thinks they will take the most time to write the alphabet forward? [52 seconds or 1 letter every 2 seconds] Alright, let's get up and stand in order from fastest predicted time to slowest predicted time."

Learners order themselves

"As I listened in, it became apparent that several of you made similar predictions. It would be interesting to see how the predictions cluster. But we could potentially have a lot of unique guesses. In order to gather those guesses, let's round our predictions to the nearest quarter-of-a-minute. For example, Sam guessed 20 seconds forward and 55 seconds backwards. He would round to 1/4 of a minute for forward and one minute, four-fourths, backwards. Work with your neighbors to round your predictions to the nearest quarter-of-a-minute and then post them on the board - forward at the front and backwards at the back."

Learners post rounded predictions

"What do you notice about the data sets?" [The writing in reverse predictions are "higher" and more spread out than the forward predictions.] 

"Why?" [We are familiar with writing the alphabet forwards so we think we can do it faster and know more what to expect.]

Activity - Writing the Alphabet in Reverse Order

Directions: "I am going to give you three pieces of paper."

At this point in my lesson, one of the pre-service teachers asked, "We're really going to get to do it, aren't we? We're really going to find out how long it takes us to write the alphabet from Z to A? Is it weird that I am so excited about this?" I reassured her that it wasn't weird - that my other pre-service teachers had designed a pretty cool lesson.

Directions continued: "You have a choice. On the yellow paper, you may write the alphabet forward on one side and use it to help you to write it from Z to A on the other side. You'll see the second sheet has the alphabet already on the back in the form of classic blocks, like the ones my grandson plays with. If you choose that one, you will incur a 1/2 minute penalty, which means you will add 30 seconds to your time. The last piece is simply scrap paper; use it if you want to try to write the alphabet from Z to A without any other support.

"A few more things: 

• You must start at Z and write the letters in reverse order to A. You can't cheat and start at A on the right-side of your paper. 
• The letters must be legible. Your table-mates will decide if they can read your letters, and you will earn a 5 second penalty for each letter they can't read.
• When you finish, check the timer on the front board, record your time, and round it to the nearest quarter-of-a-minute.

At this point, a student rose his hand to ask a question. The girl who was so excited blurted out, "I just want to get started!" The other student asked if the letters had to be upper or lower case. I said it didn't matter to me.

Set the online stopwatch and say, "Go!"

When everyone is finish, have learners trade papers check letters for legibility.

Reflection - Noticing and Naming

Directions: "If you used the yellow paper (wrote A to Z on the back), write your result, to the nearest quarter-of-a-minute, on the yellow sticky note. If you used the blocks, and added 30 seconds to your time, write your rounded result on the blue sticky note. If you did it without any support, write your rounded result on the pink sticky note. Your rounded results go on the line plot on the back board underneath your predictions."

"What do you notice?" [Look for opportunities to introduce terminology related to measures of center and spread, like median, mode, and range]

I want to ... - Choice

Directions: "What do you want to do now? Here are some ideas:

• Try it again using a different level of support and add it to the line plot;
• See if there is a difference between writing in upper and lower case;
• Try it forward and compare it with your prediction;
• Gather more data from your friends and family over Thanksgiving;
• Consider other activities that ask people to do familiar things in unfamiliar ways and what the data might show; or
• Come up with your own idea to extend your learning."

What's the hurry?

The moment you (some of you) have been waiting for [insert drumroll] ... the Carousel Lesson Design process. Previously, we learned about SAFARI lessons and prototyping. In this post, I share how to encourage teachers to embrace creativity and connectivity while collaborating on a week long unit design.

First, you need some ingredients. It's best if you have: 

Investigations Curriculum

• 5 willing teachers;
• 1 set of targets;
• 1 rich curriculum;
• 5 pieces of easel paper;
• Various scented (optional), colored markers;
• Multiple sticky notes;
• 1 lesson design framework; and
• 1 timer

Each teacher is assigned one of five sequential lessons and given 5 minutes (no more, no less) to look through the lesson in order to determine what is important.  At the end of this time, they use another 2 minutes to set up the SAFARI lesson framework on their easel paper and write down some of the most important ideas from the lesson they were assigned.

After 2 minutes the teachers rotate (like a Carousel) to the next lesson. Day 1 goes to Day 2 ... and Day 5 goes to Day 1. They use what they know from their own lesson and the important points the previous teacher wrote down to inform them about the lesson. They also have exactly 2 minutes to add to the lesson. I am constantly reminding them, "Don't worry about designing it perfectly. You don't even know for sure what the lesson is about. Don't worry about offending the teacher that started the lesson. They spent all of 2 minutes on it so far."

The teachers aren't always crazy about the artificial time crunch. However, it helps to contribute to their creativity (think MacGyverMath) while ensuring progress. It keeps them from letting their perfectionism get in the way.

Rotate! And repeat ... three more times (Note: only two interactions shown below) at 2 minutes a piece.

The teachers are now back at their original lessons. They take 1 minute to read through what has been added to their initial ideas. The sticky notes are used to identify questions for the author or indicate likes (thumbs up). The teachers can also continue to add new ideas based on what they have seen in the other lessons. After 1 minute the teachers rotate again and again and again and again and again. At each lesson they answer questions, add stickies, or contribute ideas.

At the end, the teachers have spent 20 minutes to design a five-day unit.

Yes, there is still some work to do to sift out the essential elements of the lesson. These will be written in the SAFARI format and then shared with their peers for feedback. Finally, the lessons are tested out in the classroom. The next post is about one of those lessons.

Where are we in the SA F A R I?

I might have gotten a bit ahead of myself in the last post (or perhaps I am building suspense - you decide). Before I introduce you to the Carousel Lesson Design process, let me explain a bit more about the SAFARI prototypes. This should put the framework into a clearer context.

As I said, SAFARI is based on an instructional approach called the workshop model. SAFARI is an acronym for the components of the model [Schema Activation, Focus, Activity, Reflection, and "I want to ..."], and in Swahili it means journey. So in designing a lesson, we are thinking about it as a journey from the known to the new.

One thing that design thinking has shown me, is that this journey also reflects the flair and focus necessary for innovative thinking.

The Schema Activation begins with a flair. Sometimes referred to as the anticipatory set, it ought to be an open-ended invitation for everyone to join the journey. Next, the lesson quite literally Focuses the learners' attention on what to look for during the journey; this might be a "think aloud" in typical workshop lessons. Entering the Activity portion, the lesson once again flairs - allowing each learner room to roam. Here you might encounter what Dr. Jo Boaler refers to as a "low floor, high ceiling" task. After a set amount of time exploring, we refocus by Reflecting on what was important during the activity. We should not wait until everyone is finished before making time to consolidate our thinking. In fact, we want students to cry out for more time; it's what Ellin Keene calls fostering learning lust. Finally, we ask learners to consider what comes next by brainstorming "I want to ..." statements related to the lesson. Perhaps they want to spend more time exploring the task they didn't finish. This represents the final flair until tomorrow when the journey starts all over again.

After explaining the components to my teachers, I give them 10 minutes to develop three different SAFARI lessons related to some topic.

At the end of the 10 minutes, I ask the teachers to share their prototypes with a peer for feedback. The teachers are often resistant to share unfinished products because of the implicit need for perfection usually associated with typical lesson plans. I remind them, however, that they had, on average, three-and-a-third minutes on each prototype. So no one expects their lessons to be perfect.

Afterwards, the teachers express appreciation for the process. Not only have they outlined three possible lessons that they could use in their classrooms, but because the lessons were incomplete their peers were able to add innovative ideas in the blank-spaces. "I wouldn' have thought of doing it that way," one teacher admitted. She continued, "And if I had created a full-blown lesson plan, I don't think [the other teacher] would have been able to see and share this amazing idea."

It's a good reminder that the creative process is often about finding and cultivating the cracks that allow new ideas to grow. So how does this get any better? Here's what you've been waiting for - the Carousel Lesson Design ... in the next post.

Wanna go on a SA F A R I?

Recently, I have been considering how to apply principles from Design Thinking to education. For example, I think creating lesson prototypes instead of lesson plans can help teachers in several ways. 

d.school

1. We make prototypes faster than plans, which means more time for other teachery-things; 
2. Because less time is spent developing a lesson prototype, the teacher is usually less invested in sticking to it when things go poorly;
3. Multiple prototypes are often made, so if the teacher wants to shift gears, there's another idea available; and 
4. A prototype has more blank-space (less teacher control), which leaves more room for interesting interpretations of the lesson by students.

When it came time to develop a framework for the lesson prototype, I returned to a familiar instructional approach: Math Workshop. I wrote about this approach with some GVSU math colleagues in a Mathematics in the Middle School article. Table 1 from the article, shown below, gives a good overview of the Math Workshop Model that we use.

I tend to use "Schema activation" instead of  "Making connections" for the first part of the lesson because of its use in reading workshop - where I was introduced to the approach.

Last year, I shared this method with Kristin Frang. We were working on a project that required designing lessons. I wrote the framework on the board using only the initials:

Kristin said, "If only you had an "I" it could be safari."

Having been on an actual safari (and being a bit obsessive about acronyms), I couldn't believe I had never seen this before. All the framework needed was an "I" to complete the word, safari. 

The "I" became "I want to ..." as a way to recognize the importance of students making their own choice about what to explore in their learning journey. And that's the coolest thing about SAFARI lesson design - in Swahili, safari literally means travel or journey.

With the framework developed, the next thing to do was create a process for using the framework to design the lesson prototypes. I wanted to stay true to Design Thinking and use a process that would foster innovation. So in the next post, we will explore Carousel Lesson Design.

Should I keep these math flashcards?

I am working on a project with Alyssa Boike called MacGyver Math. Before I impose my kind of crazy on that site, however, I want to write a prototype post. Here it goes...

=====

Dear MacGyver Math,

I am a new second-grade teacher. Over the summer I went through some of the boxes the previous teacher left in my classroom and found a bunch of math flashcards. It's my understanding that addition, subtraction, multiplication, and division flashcards are bad because they reinforce the belief that being good at math means being fast. And who can forget those awful Around the World games in math class where one person dominated while the rest of us just sat there?

So, should I keep the flashcards or throw them away?

Sincerely,

Enlightened Elementary Educator in Elmira

***

Dear Enlightened,

Thanks for your letter. It reminded me of one of my favorite Macgyver quotes. 

While you might not want to use the flashcards to reinforce false beliefs about doing math, they might serve other purposes.

For example:

We could ask our students to sort the flashcards; "Which facts do you know and which facts are you still learning?" Then we could see if there are any known facts that they could use to help them learn the unknown facts.

Students could use an interesting/appropriate subset of flashcards to create a real graph of the answers. They could analyze the graph looking for patterns, making conjectures, and testing the conjectures. Like, "I think that if we keep sorting out our flashcards, 27 will show up the most because that's the largest product in our set of cards."

We could use the flashcards to create Which One Doesn't Belong [WODB] scenarios. Or have students create their own WODB to challenge their peers. (See the WODB blog and the book by Christopher Danielson for more information about this instructional approach.)

Finally, if we want to play a game that's not Around the World, maybe we play Go Fish. (Make up your own rules depending on what you want student to experience. Or better yet, maybe have them make the rules if you want to encourage them to play with math.)

What do you think, regular (irregular, and new) MacGyver Math readers? How might Enlightened Elementary Educator in Elmira step back and take a look at what she's got (flashcards) in a totally different way? As always, leave your suggestions in the comments.

Thanks,

MacGyver Math

=====

If you have suggestions for Enlightened in Elmira regarding using flashcards in novel ways, please address your comments to her. If you want to comment on this MacGyver Math format, please direct your comments to Dave. Either way, thanks for your participation.

How does a mathematician see the world?

And how does a math teacher help learners to see the world through a mathematician's eyes?

This was a major challenge during the past semester in my Intermediate Algebra sections. Many of the students came to class expecting me to show them a procedure that they would practice until test time - when they would reproduce the procedure and promptly forget it. Nearly all of the students had seen the Intermediate Algebra content in high school (linear, quadratic, and exponential functions) but it hadn't stuck.

This was the problem. They had seen the content. Now it was time for them to use it to see the world. So I shared pictures and videos and asked them to look at them through a mathematical lens.

At one point, a student said, "Just once, I wish I could see the world through your eyes." Exactly! Unfortunately, they were often so afraid of making a mistake, of breaking the mathematical glasses, that they were not willing to even put them on. They did not know how to be playful with the math we were exploring.

So I introduced them to Yes, And ...; this is a problem finding activity that I developed using a well-know improv game where participants accept and build on the ideas of their partners. Here are the instructions for my version:

• Provide a mathematical context (often pictures or videos) but without any identified problem;
• Pair up the students and find a fun way to identify Student A;
• Student A picks one of the contexts and finds a problem to solve;
• For one minute (this is usually enough time to get started without completely solving the problem), Student A talks through his or her thinking while Student B writes as much as possible down on a piece of paper;
• After one minute, the students switch roles. I say, "Yes, and ...," to signal the switch and to emphasize that Student B ought to build on the work that was already done.
• Student B thinks out loud for one minute while Student A records the thinking on the same paper.
• After one minute, I say, "Yes, and ...," and the roles reverse again.

Yes, And ... can go on as long as the teacher wants. I found six minutes (three rounds) to be about right the first time we played the game. One student submitted this to demonstrate her engagement with the task.

I cannot claim for certain that Yes, And ... changed my students' view of mathematics or helped them to see the world through a mathematical lens. What I know is that for six minutes they played with math. It's a start.